 Department Homepage
The College of Arts & Sciences
Department Homepage
The College of Arts & Sciences
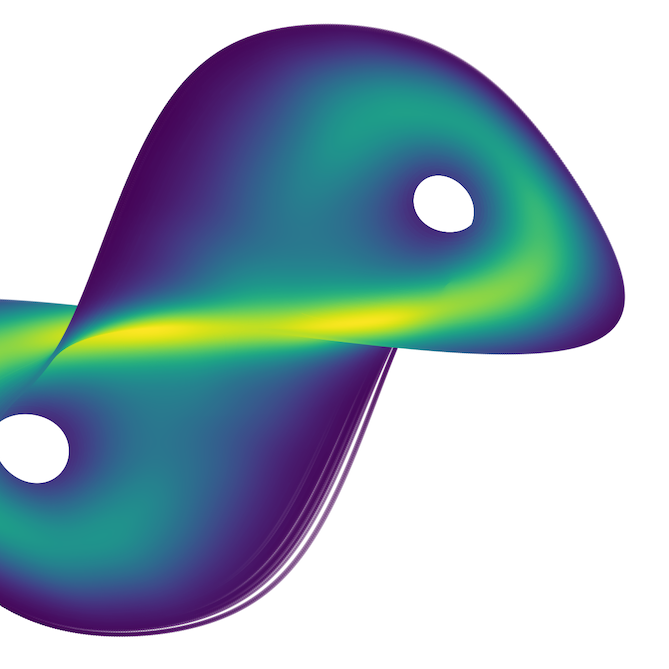
Shapes of Mathematical Elegance
"In mathematics, somehow the truth often appears beautiful. It’s nice to have something where beauty and the truth usually coincide.”




